The Third Law of Galactic Rotation

Flat rotation curves were the first clear evidence that the dynamics of galaxies do not follow the same rules as planetary systems. But they do follow rules. These include asymptotic flatness, Tully-Fisher, the luminosity-size-rotation curve shape relation (aka the `universal‘ rotation curve), Renzo’s rule, and the central density relation.

vrsd_pop — Rotation curves color coded by the characteristic surface density of stars and gas, ranging from low surface brightness galaxies (blue) to those of high surface brightness (red).

These various relations sound like a hodge-podge of random astronomical effects. This is misleading. There is a great deal of organization in the data. The surface density of stars and gas, and the acceleration (g_bar) determined by their gravitational potential, plays a defining role. Indeed, the known relations are all manifestations of a single, more fundamental relation, the radial acceleration relation.

rar — The radial acceleration relation. The centripetal acceleration measured by the rotation curve (g_obs) correlates with that predicted by the observed distribution of stars and gas (g_bar). The data consist of 2,693 resolved points along the rotation curves of 153 rotating galaxies from the SPARC database. All galaxies fall along the same relation, within the uncertainties. Red squares are binned data. The lower panel shows residuals from a fit to the data. The dashed lines are the scatter in the data; the red lines are the amount of scatter expected from measurement uncertainties.

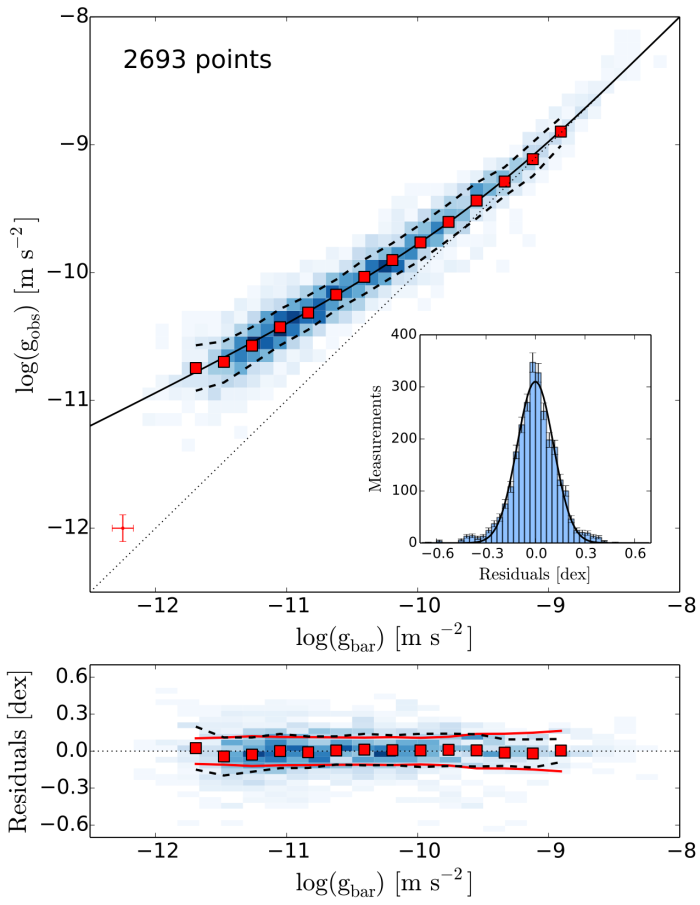
The radial acceleration relation connects what you see in galaxies with what you get for the gravitational force. This would be a trivial statement if galaxies behave as planetary systems do. They would follow the 1:1 dotted line in the figure. Instead, they bend away from that line.

Indeed, the data are consistent with a single effective force law, which can be written

rareqn

Other functional forms could also work. But they would all necessarily have a critical acceleration scale g_† ≈ 10^-10 m s^-2. This is an important scale that is ubiquitous in extragalactic astronomy. It seems to be a new fundamental scale in physics.

The critical acceleration marks the onset of the missing mass problem. Above this scale, there is no need for dark matter. Below it, the difference between the 1:1 line and the data is what we attribute to dark matter. The more the observed acceleration exceeds that which can be explained by the stars and gas, the larger the mass discrepancy.

Irrespective of interpretation, the data establish the radial acceleration relation in a purely empirical way. There is nothing but data here. The axes are independent: one is measured from rotation curves, the other from photometry. These need not be well connected – the dark matter could cause any sort of acceleration independently of the stars and gas. But they are intimately coupled.

There are no deviations from the radial acceleration relation beyond those attributable to experimental error. The residuals do not correlate with mass, size, surface brightness, color, environment, how many intelligent civilizations a galaxy hosts, or anything else. The scale that matters is not luminosity or halo mass or size. It is the acceleration determined from the surface density of stars and gas.

The radial acceleration relation is a fundamental relation. In effect, it is a law of nature. Third in our counting, but first in importance, as both flat rotation curves and the Tully-Fisher relation follow from it. It must be explained by any theory that claims to provide a satisfactory description of galaxy dynamics.