A surprising and ultimately career-altering result that I encountered while in my first postdoc was that low surface brightness galaxies fell precisely on the Tully-Fisher relation. This surprising result led me to test the limits of the relation in every conceivable way. Are there galaxies that fall off it? How far is it applicable? Often, that has meant pushing the boundaries of known galaxies to ever lower surface brightness, higher gas fraction, and lower mass where galaxies are hard to find because of unavoidable selection biases in galaxy surveys: dim galaxies are hard to see.
I made a summary plot in 2017 to illustrate what we had learned to that point. There is a clear break in the stellar mass Tully-Fisher relation (left panel) that results from neglecting the mass of interstellar gas that becomes increasingly important in lower mass galaxies. The break goes away when you add in the gas mass (right panel). The relation between baryonic mass and rotation speed is continuous down to Leo P, a tiny galaxy just outside the Local Group comparable in mass to a globular cluster and the current record holder for the slowest known rotating galaxy at a mere 15 km/s.

At the high mass end, galaxies aren’t hard to see, but they do become progressively rare: there is an exponential cut off in the intrinsic numbers of galaxies at the high mass end. So it is interesting to see how far up in mass we can go. Ogle et al. set out to do that, looking over a huge volume to identify a number of very massive galaxies, including what they dubbed “super spirals.” These extend the Tully-Fisher relation to higher masses.

Most of the super spirals lie on the top end of the Tully-Fisher relation. However, a half dozen of the most massive cases fall off to the right. Could this be a break in the relation? So it was claimed at the time, but looking at the data, I wasn’t convinced. It looked to me like they were not always getting out to the flat part of the rotation curve, instead measuring the maximum rotation speed.
Bright galaxies tend to have rapidly rising rotation curves that peak early then fall before flattening out. For very bright galaxies – and super spirals are by definition the brightest spirals – the amplitude of the decline can be substantial, several tens of km/s. So if one measures the maximum speed instead of the flat portion of the curve, points will fall to the right of the relation. I decided not to lose any sleep over it, and wait for better data.
Better data have now been provided by Di Teodoro et al. Here is an example from their paper. The morphology of the rotation curve is typical of what we see in massive spiral galaxies. The maximum rotation speed exceeds 300 km/s, but falls to 275 km/s where it flattens out.

Adding the updated data to the plot, we see that the super spirals now fall on the Tully-Fisher relation, with no hint of a break. There are a couple of outliers, but those are trees. The relation is the forest.
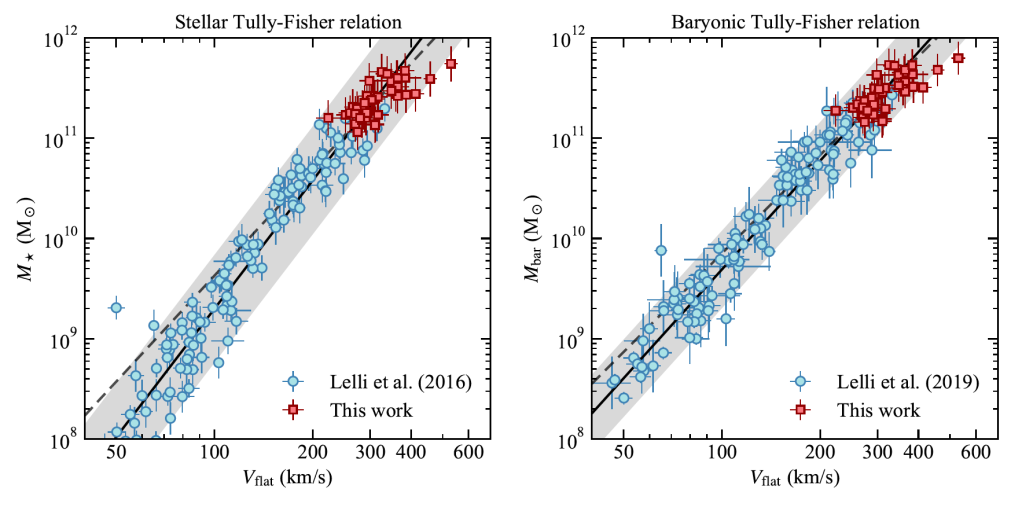
That’s a good plot, but it stops at 108 solar masses, so I couldn’t resist adding the super spirals to my plot from 2017. I’ve also included the dwarfs I discussed in the last post. Together, we see that the baryonic Tully-Fisher relation is continuous over six decades in mass – a factor of million from the smallest to the largest galaxies.

The strength of this correlation continues to amaze me. This never happens in extragalactic astronomy, where correlations are typically weak and have lots of intrinsic scatter. The opposite is true here. This must be telling us something.
The obvious thing that this is telling us is MOND. The initial report that super spirals fell off of the Tully-Fisher relation was widely hailed as a disproof of MOND. I’ve seen this movie many times, so I am not surprised that the answer changed in this fashion. It happens over and over again. Even less surprising is that there is no retraction, no self-examination of whether maybe we jumped to the wrong conclusion.
I get it. I couldn’t believe it myself, to start. I struggled for many years to explain the data conventionally in terms of dark matter. Worked my ass off trying to save the paradigm. Try as I might, nothing worked. Since then, many people have claimed to explain what I could not, but so far all I have seen are variations on models that I had already rejected as obviously unworkable. They either make unsubstantiated assumptions, building a tautology, or simply claim more than they demonstrate. As long as you say what people want to hear, you will be held to a very low standard. If you say what they don’t want to hear, what they are conditioned not to believe, then no standard of proof is high enough.
MOND was the only theory to predict the observed behavior a priori. There are no free parameters in the plots above. We measure the mass and the rotation speed. The data fall on the predicted line. Dark matter models did not predict this, and can at best hope to provide a convoluted, retroactive explanation. Why should I be impressed by that?
I have opened comments again, against my better judgement. Last time someone abused the terms of service by posting long, repeated rants about some random crackpot theory. Don’t do that.
LikeLike
I’ll take the opportunity to say that reading your blog has educated me about MOND. I’m just an interested amateur, and before I started reading your blog what little opinion of MOND I had was that it seemed a fringe idea, maybe even a crackpot one. Your blog makes it clear it’s, at worst, a serious contender we should be studying, and at best it might be The Truth.
So great blog and thankyou for it!
LikeLiked by 1 person
Thanks. Yes, that’s what I thought too, initially. The more I learned, the more I realized it wasn’t so easily dismissed. Unfortunately, most of the relevant scientific community remains entrenched in the phase “surely I don’t need to learn about this.”
LikeLiked by 1 person
As always, great work Stacy.
I agree that those long rant posts are really annoying. They totally infringe on my territory. 🙂
Anyway, what are the possibilities of the James Webb telescope providing useful data for testing MoND and/or Dark Matter? I.E. will the Infrared data be significant on its own, or will it mainly just confirm data from the visible spectrum?
LikeLike
The Tully-Fisher relation is entitled to be upgraded to the “Tully-Fisher law” now (with Nobel Prize attached perhaps). The James-Webb telescope might discover new empirical details and constraints at the margins, but it won’t undo the important and substantive empirical result graphically described here; a result that also puts important constraints on how galaxies actually must grow and evolve with age, and helps invalidate the need for any form of unobservable Dark Matter (or other “hidden variables” surplus to those required by MOND) acting over observable galactic size scales and time scales.
LikeLike
Yes, I think TF is a de facto law of nature, along with Sancisi’s law, the central density relation, and the radial acceleration relation (basically Milgrom’s law). Regardless of the physics driving these relations, they are a fundamental aspect of observed reality in the same sense as Kepler’s laws. And of course this list should include flat rotation curves… I often hear people complain that Vera Rubin wasn’t awarded the Nobel Prize for discovering dark matter, but part of the problem there is that that’s too broad a claim. The discovery of mass discrepancies can be attributed to many people, going back to at least the 1930s in the work of Oort and then Zwicky. Establishing that rotation curves are inevitably flat as a new natural law was more than enough in itself.
LikeLike
Hi Stacy,
Thank you for this post on where super-spirals lie in the baryonic Tully-Fisher relation.
In your second diagram I can see the 6 high rotators (blue dots) from Ogle et al that lie well to the right of the Tully-Fisher relation. And I can see that these have moved to the left in the Di Teodoro diagram because, as you mention, the flat part of their rotation curves has been reassessed to lower values. Once corrected these super-spirals now lie on the Tully-Fisher line.
But in your second diagram I can also see the yellow dots of Catinella et al; many of these appear to be slow rotators and lie well to the left of the Tully-Fisher relation. What has happened to these galaxies as they were not covered by Di Teodoro and so are missing from the Di Teodoro diagram? – they have slightly lower masses and may not qualify as super-spirals. Is it simply that the measured rotation curves are still rising and the flat part has not been reached leading to a rotation speed that is far too low? Or are the error bars (not shown) huge? Or is there really a bunch of slow rotating super-spirals?
LikeLiked by 1 person
That’s an interesting question. I looked at the preprint for the Catinella and Cortese (2015) paper [Arxiv: 1410.7464] and it says:
“Unfortunately, HI astronomy lags behind in this respect. Due to sensitivity of current instruments, as well as man-made radio interference, HI observations are still struggling to detect the weak 21 cm emission beyond z ∼0.16”
Their five observing runs at Arecibo were z=0.09-0.18, 0.09-0.25, 0.16-0.25, 0.16-0.25 and 0.16-0.32. so it looks like most of their data is coming from the the area where detections themselves are uncertain. They failed to detect HI from 10 galaxies, 9 where z=0.23-0.26 and one with z=0.17; they got HI detections from 39 galaxies out of 49 with 0.17<z<0.25.
We really don't know with the data as it is if what we are seeing is signal from hydrogen gas that isn't far enough from the centre of the galaxies to be at the constant rotation speed.
LikeLiked by 1 person
Don’t know, don’t care. After a quarter century of fact-checking these kinds of results, I’m done with sorting out other people’s mistakes. Not my problem.
LikeLiked by 1 person